Tee (T) Section Calculator
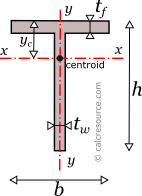
This tool calculates the properties of a T section. Enter the shape dimensions h, b, tf and tw below. The calculated results will have the same units as your input. Please use consistent units for any input.
h = | |||
b = | |||
tf = | |||
tw = | |||
Geometric properties: | |||
Area = | |||
Perimeter = | |||
yc = | |||
Properties related to x-x axis: | |||
Ix = | |||
Sx = | |||
Zx = | |||
ypna = | |||
Rgx = | |||
ADVERTISEMENT | |||
Properties related to y-y axis: | |||
Iy = | |||
Sy = | |||
Zy = | |||
Rgy = | |||
Other properties: | |||
Iz = | |||
 |
ADVERTISEMENT
Definitions
Table of contents
Geometry
The area A and the perimeter P of a tee cross-section, can be found with the next formulas:
The distance of the centroid, from the top edge, can be calculated if we consider that the first moment of area (also called static moment) of the entire T-section should be equal to the combined static moments of the web and the flange:
We have a special article about finding the centroid of a compound area. If you need more details about the procedure, get it here.
Moment of Inertia
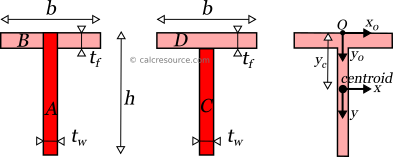
The moment of inertia of a tee section can be found, if the total area is divided into two, smaller ones, A, B, as shown in figure below. The final area, may be considered as the additive combination of A+B. Therefore, the moment of inertia of the tee section, relative to non-centroidal x0 axis, is determined like this:
where , the tee height, , the width of the flange, , the thickness of the flange (parallel to x-x) and , the thickness of the web (perpendicular to x-x).
Knowing and , the moment of inertia around the centroidal x axis, can be determined, using the Parallel Axes Theorem:
The moment of inertia of the tee section, around centroidal y axis, can be found directly, by the additive combination of C+D sub-areas:
The moment of inertia (second moment or area) is used in beam theory to describe the rigidity of a beam against flexure. The bending moment M applied to a cross-section is related with its moment of inertia with the following equation:
where , is the Young's modulus, a property of the material, and , the curvature of the beam due to the applied load. Therefore, it can be seen from the former equation, that when a certain bending moment M is applied to a beam cross-section, the developed curvature is reversely proportional to the moment of inertia .
The polar moment of inertia, describes the rigidity of a cross-section against torsional moment, likewise the planar moments of inertia described above, are related to flexural bending. The calculation of the polar moment of inertia around an axis z-z (perpendicular to the section), can be done with the Perpendicular Axes Theorem:
where the and are the moments of inertia around axes x-x and y-y that are mutually perpendicular with z-z and meet at a common origin.
The dimensions of moment of inertia are .
Elastic modulus
The elastic section modulus , of any cross section, around an axis x-x that is centroidal, describes the response of the section under elastic flexural bending, around the same axis. It is defined as:
where the moment of inertia of the section around x axis and the offset from centroid, of a section fiber, parallel to x-x axis. Typically the most distant fiber is of interest. For the T-section, the elastic section modulus , around the x-x axis, is not the same for a top-end and a bottom-end fiber. Since the T-section is not symmetric around x-axis, the distances of the two end fibers (top and bottom), from this axis, are different. The bigger Y results in the smaller , which is usually preferable for the design of the section. Therefore:
where the “min” designation is based on the assumption that , which is valid for any tee section.
For the section modulus , around y-y axis, which, for the T section, happens to be an axis of symmetry, the section modulus is found by the following formula:
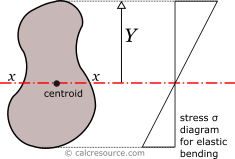
If a bending moment is applied around axis x-x, the section will respond with normal stresses, varying linearly with the distance from the neutral axis (which under elastic regime coincides to the centroidal x-x axis). Over neutral axis the stresses are zero. Absolute maximum , will occur at the most distant fiber, with magnitude given by the formula:
From the last equation, the section modulus can be considered for flexural bending, a property analogous to cross-sectional A, for axial loading. For the latter, the normal stress is F/A.
The dimensions of section modulus are .
Plastic modulus
The plastic section modulus is similar to the elastic one, but defined with the assumption of full plastic yielding of the cross section, due to flexural bending. In that case, the whole section is divided in two parts, one in tension and one in compression, each under uniform stress field. For materials with equal tensile and compressive yield stresses, this leads to the division of the section into two equal areas, in tension and in compression, separated by the neutral axis. This axis is called plastic neutral axis, and for non-symmetric sections, is not the same with the elastic neutral axis (which again is the centroidal one). The plastic section modulus is given by the general formula:
where the distance of the centroid of the compressive area from the plastic neutral axis and the respective distance of the centroid of the tensile area .
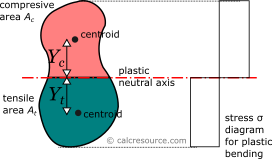
Around x axis
For the case of a T section, under x-x bending, the position of the plastic neutral axis can be found by either one of the following two equations:
which becomes:
where the distance of the plastic neutral axis from the top edge of the flange. The first equation is valid when the plastic neutral axis passes through the web, while the second one becomes valid when the axis passes through the flange. Generally, it can't be known which equation is relevant beforehand.
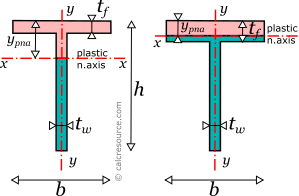
Once the plastic neutral axis is determined, the calculation of the centroids of the compressive and tensile areas becomes straightforward. For the first case, that is when the axis crosses the web, the plastic modulus can be found like this:
which can be simplified to:
For the second case, that is when the axis passes through the flange, the plastic modulus is found with equation:
which becomes:
Around y axis
For y-y bending, the plastic neutral axis passes through centroid (due to the symmetry). The calculation of the plastic modulus can be easily formulated:
Radius of gyration
Radius of gyration Rg of a cross-section, relative to an axis, is given by the formula:
where I the moment of inertia of the cross-section around the same axis and A its area. The dimensions of radius of gyration are . It describes how far from centroid the area is distributed. Small radius indicates a more compact cross-section. Circle is the shape with minimum radius of gyration, compared to any other section with the same area A.
Tee section formulas
The following table, lists the formulas, for the calculation the main mechanical properties of a T section.
Tee (T) section formulas | |
|---|---|
| Quantity | Formula |
| Area: | |
| Perimeter: | |
| Centroid: | |
| Moments of inertia | |
| Elastic modulus: | |
| Plastic modulus: | |
Plastic neutral axis: (distance from top) | |
where: | |