ADVERTISEMENT
Definitions
Geometry
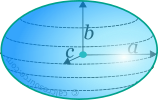
The ellipsoid is a 3-dimensional surface, the points of which, in Cartesian coordinates, satisfy the following equation:
where a,b,c are the radii of the ellipsoid along its principal axes x, y and z respectively. It can be seen from the above formula, that ellipsoid is, in three dimensions, analogous to the ellipse in two dimensions.
The volume enclosed by an ellipsoid is given by the formula:
where a, b, c the three radii along the ellipsoid principal axes.
The outer surface area of the ellipsoid is not available in a closed form formula. Exact calculation requires the evaluation of elliptical integrals of the first and second kind, and is given by the equation:
where the incomplete elliptic integral of the first kind, the incomplete elliptic integral of the second kind and:
An approximate formula for the estimation of the surface area of the ellipsoid is:
A value of p=1.6075 yields the best approximation, with an error of the order 1%.
The calculator in this page finds the surface of the ellipsoid, using the precise formula, through numerical computation of the elliptic integrals.